Arithmetic – MarkUp, Discount, Profit

Mark Up, Discount and Profit questions confuse a lot of people. But, actually, most of them are absolute sitters — very easy to solve — a free ride! How? We will just see. Let me begin with the previous post’s question.

Question: If a retailer marks up an article by 40% and then offers a discount of 10%, what is his percentage profit?

Let us say the retailer buys the article for €100 (€100 is his cost price of the item). He marks it up by 40% i.e. increases his cost price by 40% () and puts a tag of €140 on the article. Now, the article remains unsold and he puts it on sale – 10% off everything. So the article marked at €140, gets €14 off and sells at €126 (because ). This €126 is the selling price of the article. To re-cap, we obtained this selling price in the following way:

Cost Price  (1 + Mark Up%)  (1 – Discount%)  Selling Price

The profit made on the item is €26 (obtained by subtracting 100, the retailer’s cost price, from 126, the retailer’s selling price).

He got a profit % of % 

Or we can say that Cost Price  (1 + Profit%) =  Selling Price

The above shows the two ways in which you can reach the selling price: using mark-up and discount or using profit. The same thing is depicted in the diagram below:

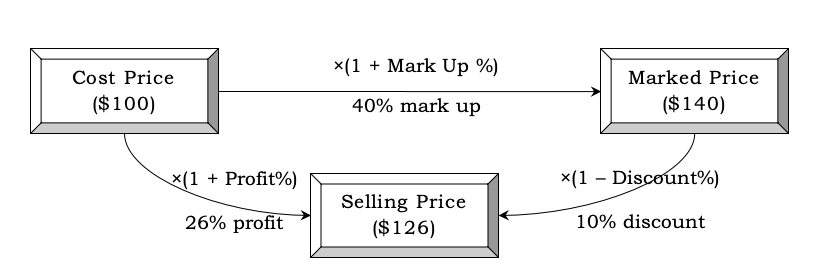
Therefore, Cost Price  (1 + Mark Up%)  (1 – Discount%)= Cost Price  (1 + Profit%)

Or

(1 + Mark Up%)  (1 – Discount%)= (1 + Profit%)

Look at the numbers here: Mark Up: 40%, Discount: 10%, Profit: 26% (Not 30% that we might expect because 40% – 10% = 30%)

Why? Because the discount offered was on €140, not on €100. So a bigger amount of €14 was reduced from the price. Hence the profit decreased. This leads us to an extremely important question in percentages – What is the base? 100 was increased by 40% but the new number 140 was decreased by 10%. So in the two cases, the bases were different. Hence, you cannot simple subtract 10 from 40 and hope to get the Profit %. Also, mind you, almost certainly, 30% will be one of the answer choices, albeit incorrect. (The GMAT doesn’t forego even the smallest opportunity of tricking you into making a mistake!)

Let’s see this concept in action on a tricky third party question:

A dealer offers a cash discount of 20%. Further, a customer bargains and receives 20 articles for the price of 15 articles. The dealer still makes a profit of 20%. How much percent above the cost price were his articles marked?

a) %

b) %

c) %

d) %

e) %

This question involves two discounts:

1. the straight 20% off

2. discount offered by selling 20 articles for the price of 15 – a discount of cost price of 5 articles on 20 articles i.e. a discount of %

Using the formula given above:

m = 100

Therefore, the markup was 100%.

Answer (A).

Note: The two discounts are successive percentage discounts.