Menu
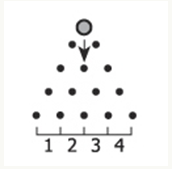
Today, let’s take a look at an official GMAT question involving visual skills. It takes a moment to understand the given diagram, but at close inspection, we’ll find that this question is just a simple probability question – the trick is in understanding the symmetry of the figure:
Question: The figure shown represents a board with 4 rows of pegs, and at the bottom of the board are 4 cells numbered 1 to 4. Whenever the ball shown passes through the opening between two adjacent pegs in the same row, it will hit the peg directly beneath the opening. The ball then has the probability 
of passing through the opening immediately to the left of that peg and probability of passing through the opening immediately to the right. What is the probability that when the ball passes through the first two pegs at the top it will end in cell 2?
(A) 
(B) 
(C) 
(D) 
(E)
First, understand the diagram. There are small pegs arranged in rows and columns. The ball falls between two adjacent pegs and hits the peg directly below. When it does, there are two ways it can go – either to the opening on the left or to the opening on the right. The probability of each move is equal, i.e. 
.
The arrow shows the first path the ball takes. It is dropped between the top two pegs, hits the peg directly below it, and then either drops to the left side or to the right. The same process will be repeated until the ball falls into one of the four cells – 1, 2, 3 or 4.
Method 1: Using Symmetry
Now that we understand this process, let’s examine the symmetry in this diagram.
Say we flip the image along the vertical axis – what do we get? The figure is still exactly the same, but now the order of cells is reversed to be 4, 3, 2, 1. The pathways in which you could reach Cell 1 are now the pathways in which you can use to reach Cell 4.
OR think about it like this:
To reach Cell 1, the ball needs to turn left-left-left.
To reach Cell 4, the ball needs to turn right-right-right.
Since the probability of turning left or right is the same, the situations are symmetrical. This will be the same case for Cells 2 and 3.
Therefore, by symmetry, we see that:
The probability of reaching Cell 1 = the probability of reaching Cell 4.
Similarly:
The probability of reaching Cell 2 = the probability of reaching Cell 3. (There will be multiple ways to reach Cell 2, but the ways of reaching Cell 3 will be similar, too.)
The total probability = the probability of reaching Cell 1 + the probability of reaching Cell 2 + the probability of reaching Cell 3 + the probability of reaching Cell 4 = 1
Because we know the probability of reaching Cells 1 and 4 are the same, and the probabilities of reaching Cells 2 and 3 are the same, this equation can be written as:
2×(the probability of reaching Cell 1) + 2×(the probability of reaching Cell 2) = 1
Let’s find the probability of reaching Cell 1:
After the first opening (not the peg, but the opening between pegs 1 and 2 in the first row), the ball moves left (between pegs 1 and 2 in second row) or right (between pegs 2 and 3 in second row). It must move left to reach Cell 1, and the probability of this = .
After that, the ball must move left again – the probability of this occurring is also , since probability of moving left or right is equal. Finally, the ball must turn left again to reach Cell 1 – the probability of this occurring is, again, . This means that the total probability of the ball reaching Cell 1 = 
Plugging this value into the equation above:
probability of reaching Cell 2 = 1
Therefore, the probability of reaching Cell 2 = 
Method 2: Enumerating the Cases
You can also answer this question by simply enumerating the cases.
At every step after the first drop between pegs 1 and 2 in the first row, there are two different paths available to the ball – either it can go left or it can go right. This happens three times and, hence, the total number of ways in which the ball can travel is 2×2×2 = 8
The ways in which the ball can reach Cell 2 are:
Left-Left-Right
Left-Right-Left
Right-Left-Left
So, the probability of the ball reaching Cell 2 is .
Note that here there is a chance that we might miss some case(s), especially in problems that involve many different probability options. Hence, enumerating should be the last option you use when tackling these types of questions on the test.
