Menu
Considering a two-dimensional figure, a tangent is a line that touches a curve at a single point. Here are some examples of tangents:
In each of these cases, the line touches the curve at a single point. In the case of a circle, when you draw the radius of the circle from the center to the point of contact with the tangent, the radius is perpendicular to the tangent (as demonstrated in the figure on the right, above).
Today, we will look at a question involving a tangent to a parabola:
If 
is tangent to the 
-axis, what is the value of the positive number 
?
(A) 
(B) 
(C) 
(D)
(E) 
Let’s first try to understand what the question is saying.
is a tangent to the 
-axis. We know that the -axis is a straight line, so must be a curve. A quadratic equation, such as our given equation of 
, gives a parabola. Since the 
term in the equation is positive, the parabola would be facing upwards and touching the -axis at a single point, such as:
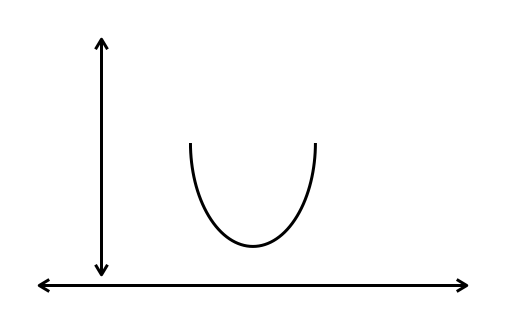
Since the parabola touches the -axis in only one point, it means the quadratic has only one root, or in other words, the quadratic must be a perfect square.
Therefore, 
To get in the form 
Note that if 
takes this value, the quadratic will have only one root.
Plugging this value of back into our equation, we will get: 
We know that the root of is the point where the value of the 
coordinate is 0. Therefore:
At this co-ordinate, the parabola will touch the axis.
[This calculation was shown only to help you completely understand the question. We could have easily stopped at 
]
Therefore, our answer is (A).
The question can be solved in various other ways – think of how!
